In a recent lecture, we were introduced to the topic of game theory and a specific type of game: “The Prisoner’s Dilemma”. In this game, the strategies that the rational players will play is not the strategy that gives the greatest payoff for both players. The article “Game Theory Explains the Pandemic”, the author Malini Sharma likens the choice to social distance during the pandemic to the Prisoner’s Dilemma.
While social distancing should be practiced with all members in a community, to demonstrate how social distancing can be a Prisoner’s Dilemma game, she sets up a two-player game and each player has two strategies: strategy “C”, which is the choice for the player to maintain social distancing and strategy “D”, which is the choice for the player to not social distance, i.e. go outside of their home. The payoff matrix in the article looks like this:
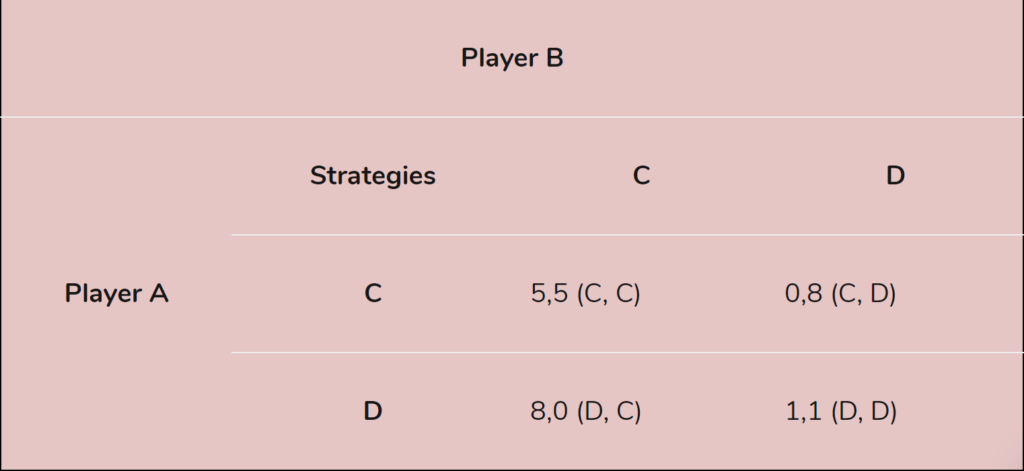
We can see that this is very similar to the payoff matrix of the Prisoner’s Dilemma as shown in class, and that the best strategy for the two players would be (C, C) with payoff (5, 5). However we can see that both players the strategy they will choose is “D”, as that is the dominant strategy for both players regardless of what the other plays. This means it is “best” for both players to not socially distance which is strategy (D, D) with payoff (1, 1). The author explains why this could possibly happen. If player A stays inside and socially distances but player B goes out, the payoff for the person who goes out is 8 and the one that doesn’t gets 0 payoff, she states that “player A will get a small benefit (8, 0) but at the cost to others in the society”. Although she doesn’t describe exactly what the payoffs are, it makes sense. The person who goes out gets to go to work and make money, or go out and enjoy themselves (which is the payoff). Meanwhile the person who doesn’t go out doesn’t really get the benefit of social distancing because of the other player breaking the rules and they do not get to go out, possibly meaning they are unable to work, or maybe their mental state is not as good due to the isolation, hence the lower payoff of 0. This means if both players choose strategy D, they get a payoff of being able to go out, but it is severely decreased as the risk of infection gets higher.
Overall, game theory could possibly explain the current state of Ontario’s COVID-19 situation. From an article from the CBC many of the people who contracted COVID are younger people and they speculate that the reasons why they break social distancing are:
- That they do not take the pandemic as seriously
- They need to go to work
Rewording this in a game theory way, we can say that for the first reason, the decrease in payoff to not social distance is not enough to deter people. The second reason would be that going to work and risking COVID-19, gives a greater payoff than staying home. Finally, the question now is how could Ontario increase the numbers of younger people social distancing. With the game theory model above they have two options:
- Decrease payoff of breaking social distancing rules (e.g. fines)
- Or increase payoff of staying home and following social distancing (e.g. financial support like CERB)
Sources:
One reply on “How Prisoner’s Dilemma and Game Theory can explain people’s behaviour during the COVID-19 lockdown.”
Although it’s pretty obvious now that I think about it, I never really considered how the Prisoner’s Dilemma would be applicable to the current pandemic so this was an interesting read.
I wonder how the payoffs would change over time. I think people are starting to get desensitized to the pandemic. They’re starting to take riskier actions than they would have at the beginning of the lockdown. In addition, would the payoffs for those who choose to go out increase the longer they choose to social distance? Would those who choose to ignore current guidelines suffer from diminishing returns? This is a really interesting situation to apply the iterated Prisoner’s Dilemma to (The Prisoner’s Dilemma is repeated except both of them remember which strategy the other previously chose). If I know a lot of people are going to the store on a certain day, maybe I’ll avoid peak hours? Maybe someone went to the store and actually caught COVID there. Guess I won’t be going near the store any time soon.
I think there’s a lot going on there and I would be greatly interested in seeing how other aspects of game theory can be applied to people’s decision-making when it comes to COVID.